Question

The city of Anvil is currently home to 21000 people, and the population has been growing at a continuous rate of 4% per year. The city of Brinker is currently home to 7000 people, and the population has been growing at a continuous rate of 5% per year. In how many years will the populations of the two towns be equal

Answer 1

They'll reach the same population in approximately 113.24 years.

Explanation:

Since both population grows at an exponential rate, then their population over the years can be found as:

`\text{population}(t) = \text{population}(0)*(1 + (r)/(100))^t`

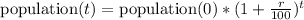
For the city of Anvil:

`\text{population anvil}(t) = 21000*(1.04)^t`

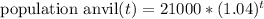
For the city of Brinker:

`\text{population brinker}(t) = 7000*(1.05)^t`

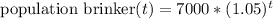
We need to find the value of "t" that satisfies:

`\text{population brinker}(t) = \text{population anvil}(t)\\21000*(1.04)^t = 7000*(1.05)^t\\ln[21000*(1.04)^t] = ln[7000*(1.05)^t]\\ln(21000) + t*ln(1.04) = ln(7000) + t*ln(1.05)\\9.952 + t*0.039 = 8.8536 + t*0.0487\\t*0.0487 - t*0.039 = 9.952 - 8.8536\\t*0.0097 = 1.0984\\t = (1.0984)/(0.0097)\\t = 113.24`

They'll reach the same population in approximately 113.24 years.