Answer:
The substance's half-life is 6.4 days
Explanation:
Recall that the half life of a substance is given by the time it takes for the substance to reduce to half of its initial amount. So in this case, where they give you the constant k (0.1088) in the exponential form:
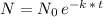
we can replace k by its value, and solve for the time "t" needed for the initial amount
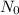 to reduce to half of its value (
to reduce to half of its value (
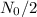 ). Since the unknown resides in the exponent, to solve the equation we need to apply the natural logarithm:
). Since the unknown resides in the exponent, to solve the equation we need to apply the natural logarithm:
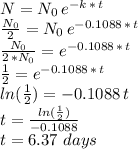
which rounded to the nearest tenth is: 6.4 days