Question

Combine and simplify the following radical expression

ASAP ASAP ASAP ASAP

Answer 1

`\sqrt[3]{3}`

Explanation:

Our expression is:
`(1)/(3) \sqrt[3]{81}`.

Let's focus on the cube root of 81 first. What's the prime factorisation of 81? It's simply: 3 * 3 * 3 * 3, or
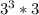`3^3*3`. Put this in for 81:

`\sqrt[3]{81} =\sqrt[3]{3^3*3}=\sqrt[3]{3^3} *\sqrt[3]{3}`

We know that the cube root of 3 cubed will cancel out to become 3, but the cube root of 3 cannot be further simplified, so we keep that. Our outcome is then:

`\sqrt[3]{3^3} *\sqrt[3]{3}=3\sqrt[3]{3}`

Now, let's multiply this by 1/3, as shown in the original problem:

`(1)/(3)* 3\sqrt[3]{3}=\sqrt[3]{3}`

Thus, the answer is
`\sqrt[3]{3}`.

~ an aesthetics lover