Question

A florist has to choose four different types of flowers to include in a bouquet. In how many ways can the florist do this, if there are 5 different types of flowers to choose from?

Answer 1

Explanation:

Given:

Type of Flowers = 5

To choose = 4

Required

Number of ways 4 can be chosen

The first flower can be chosen in 5 ways

The second flower can be chosen in 4 ways

The third flower can be chosen in 3 ways

The fourth flower can be chosen in 2 ways

Total Number of Selection = 5 * 4 * 3 * 2

Total Number of Selection = 120 ways;

Alternatively, this can be solved using concept of Permutation;

Given that 4 flowers to be chosen from 5,

then n = 5 and r = 4

Such that

`nPr = (n!)/((n - r)!)`

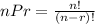
Substitute 5 for n and 4 for r

`5P4 = (5!)/((5 - 4)!)`

`5P4 = (5!)/(1!)`

`5P4 = (5*4*3*2*1)/(1)`

`5P4 = (120)/(1)`

`5P4 = 120`

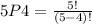
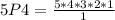
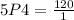
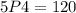
Hence, the number of ways the florist can chose 4 flowers from 5 is 120 ways