Question

The body temperatures of a group of healthy adults have a​ bell-shaped distribution with a mean of 98.19degreesF and a standard deviation of 0.61degreesF. Using the empirical​ rule, find each approximate percentage below. a. What is the approximate percentage of healthy adults with body temperatures within 3 standard deviations of the​ mean, or between 96.36degreesF and 100.02degrees​F? b. What is the approximate percentage of healthy adults with body temperatures between 96.97degreesF and 99.41degrees​F?

Answer 1

a) From the empirical rule we know that within 3 deviations from the mean we have 99.7% of the data so then that would be the answer for this case.

b)
`z=(96.97-98.19)/(0.61)=-2`

`z=(99.41-98.19)/(0.61)=2`

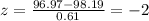
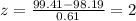
And within 2 deviations from the mean we have 95% of the values.

Explanation:

For this case we know that the distribution of the temperatures have the following parameters:

`\mu = 98.19, \sigma =0.61`

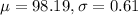
Part a

From the empirical rule we know that within 3 deviations from the mean we have 99.7% of the data so then that would be the answer for this case.

Part b

We can calculate the number of deviations from the mean with the z score with this formula:

`z=(X -\mu)/(\sigma)`

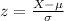
And using this formula we got:

`z=(96.97-98.19)/(0.61)=-2`

`z=(99.41-98.19)/(0.61)=2`

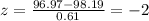
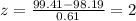
And within 2 deviations from the mean we have 95% of the values.