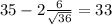Question

The mean age of employees at a large corporation is 35 years with a standard deviation of 6 years. Random samples of size 36 are drawn from this population and the mean of each sample is determined. Find the two symmetric values of the sample mean that contain the middle 95% of all sample means between them.

Answer 1

`\bar X \sim N(\mu, (\sigma)/(√(n)))`

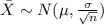
We know that from the empirical rule we know that within two deviations from the mean we have 95% of the values and if we find the limits we got:

`35 -2 (6)/(√(36))= 33`

`35 +2 (6)/(√(36))= 37`

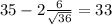
Explanation:

For this case we have the following info given:

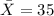`\bar X = 35` the sample mean

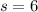`s =6` the sample deviation

`n =36` represent the sample mean

Since the sample size is higher than 30 we can use the normla approximation and we have this:

`\bar X \sim N(\mu, (\sigma)/(√(n)))`

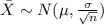
We know that from the empirical rule we know that within two deviations from the mean we have 95% of the values and if we find the limits we got:

`35 -2 (6)/(√(36))= 33`

`35 +2 (6)/(√(36))= 37`