Question

Find the coordinates of M' after a reflection of the triangle across the parallel lines; first across the line y = -3 and then across y = 2. Answer in (a, b) form. Part 9a​

Answer 1

Answer: (-3, 5)

===============================================================

Step-by-step explanation:

The first step is to locate point M. It is at (-3, -5). Ignore the other points as your teacher is not asking to transform them.

Reflecting point M over the line y = -3 will have us move 2 units up to arrive at the line of reflection, and then we move 2 more additional units up to get to N = (-3, -1).

Now reflecting point N over the line y = 2 will have us move (-3,-1) three units up to get to the line of reflection, and then we move up another 3 units to get to P = (-3,2) which is the last stop. This is also the location of point M'.

See diagram below.

--------

Extra info: Overall, those two reflections combine to a translation of shifting 10 units up. Algebraically, we can write the translation rule
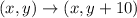`(x,y) \to (x,y+10)`. Two reflections only turn into a translation if and only if the lines of reflection are parallel.