Question

Benzoic acid is a natural fungicide that naturally occurs in many fruits and berries. The sodium salt of benzoic acid, sodium benzoate, is a common additive in food products; it is used for its ability to inhibit the growth of mold, yeast and some bacteria. Consider the titration of a 50.0 ml sample of 0.300 M benzoic acid (HC7H5O2) with 0.250 M NaOH. The ka for benzoic acid is 6.5 x 10-5

a. Calculate the pH after 20.0 mL of base has been added
b. Calculate the pH at the equivalent point (make sure to note any assumptions that have been made)
c. Calculate the pH after 100 mL of the base has been added

Answer 1

a. pH = 2.52

b. pH = 8.67

c. pH = 12.83

Step-by-step explanation:

The equation of the titration between the benzoic acid and NaOH is:

C₆H₅CO₂H + OH⁻ ⇄ C₆H₅CO₂⁻ + H₂O (1)

a. To find the pH after the addition of 20.0 mL of NaOH we need to find the number of moles of C₆H₅CO₂H and NaOH:

`\eta_(NaOH) = C*V = 0.250 M*0.020 L = 5.00 \cdot 10^(-3) moles`

`\eta_{C_(6)H_(5)CO_(2)H}i = C*V = 0.300 M*0.050 L = 0.015 moles`

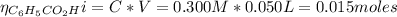
From the reaction between the benzoic acid and NaOH we have the following number of moles of benzoic acid remaining:

`\eta_{C_(6)H_(5)CO_(2)H} = \eta_{C_(6)H_(5)CO_(2)H}i - \eta_(NaOH) = 0.015 moles - 5.00 \cdot 10^(-3) moles = 0.01 moles`

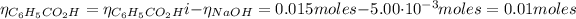
The concentration of benzoic acid is:

`C = (\eta)/(V) = (0.01 moles)/((0.020 + 0.050) L) = 0.14 M`

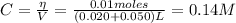
Now, from the dissociation equilibrium of benzoic acid we have:

C₆H₅CO₂H + H₂O ⇄ C₆H₅CO₂⁻ + H₃O⁺

0.14 - x x x

`Ka = ([C_(6)H_(5)CO_(2)^(-)][H_(3)O^(+)])/([C_(6)H_(5)CO_(2)H])`

`Ka = (x*x)/(0.14 - x)`

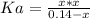
`6.5 \cdot 10^(-5)*(0.14 - x) - x^(2) = 0` (2)

By solving equation (2) for x we have:

x = 0.0030 = [C₆H₅CO₂⁻] = [H₃O⁺]

Finally, the pH is:

`pH = -log([H_(3)O^(+)]) = -log (0.0030) = 2.52`

b. At the equivalence point, the benzoic acid has been converted to its conjugate base for the reaction with NaOH so, the equilibrium equation is:

C₆H₅CO₂⁻ + H₂O ⇄ C₆H₅CO₂H + OH⁻ (3)

The number of moles of C₆H₅CO₂⁻ is:

`\eta_{C_(6)H_(5)CO_(2)^(-)} = \eta_{C_(6)H_(5)CO_(2)H}i = 0.015 moles`

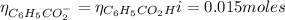
The volume of NaOH added is:

`V = (\eta)/(C) = (0.015 moles)/(0.250 M) = 0.060 L`

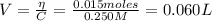
The concentration of C₆H₅CO₂⁻ is:

`C = (\eta)/(V) = (0.015 moles)/((0.060 L + 0.050 L)) = 0.14 M`

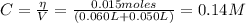
From the equilibrium of equation (3) we have:

C₆H₅CO₂⁻ + H₂O ⇄ C₆H₅CO₂H + OH⁻

0.14 - x x x

`Kb = ([C_(6)H_(5)CO_(2)H][OH^(-)])/([C_(6)H_(5)CO_(2)^(-)])`

`((Kw)/(Ka))*(0.14 - x) - x^(2) = 0`

`((1.00 \cdot 10^(-14))/(6.5 \cdot 10^(-5)))*(0.14 - x) - x^(2) = 0`

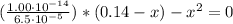
By solving the equation above for x, we have:

x = 4.64x10⁻⁶ = [C₆H₅CO₂H] = [OH⁻]

The pH is:

`pOH = -log[OH^(-)] = -log(4.64 \cdot 10^(-6)) = 5.33`

`pH = 14 - pOH = 14 - 5.33 = 8.67`

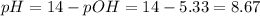
c. To find the pH after the addition of 100 mL of NaOH we need to find the number of moles of NaOH:

`\eta_(NaOH)i = C*V = 0.250 M*0.100 L = 0.025 moles`

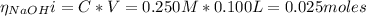
From the reaction between the benzoic acid and NaOH we have the following number of moles remaining:

`\eta_(NaOH) = \eta_(NaOH)i - \eta_{C_(6)H_(5)CO_(2)H} = 0.025 moles - 0.015 moles = 0.010 moles`

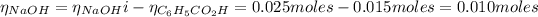
The concentration of NaOH is:

`C = (\eta)/(V) = (0.010 moles)/(0.100 L + 0.050 L) = 0.067 M`

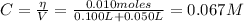
Therefore, the pH is given by this excess of NaOH:

`pOH = -log([OH^(-)]) = -log(0.067) = 1.17`

`pH = 14 - pOH = 12.83`

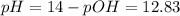
I hope it helps you!