Answer:
The approximate percentage of cars that remain in service between 73 and 84 months
P( 73 < x < 84 ) = 0.02145 = 2.1 %
Step-by-step explanation:
Step-by-step explanation:-
Given mean of the Population 'μ ' = 51 months
Standard deviation of the Population 'σ' = 11 months
Let 'X' be the random variable of Normal distribution
Let 'X' = 73
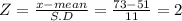
Let 'X' = 84
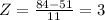
The approximate percentage of cars that remain in service between 73 and 84 months
P( 73 < x < 84 ) = P( 2 < Z < 3)
= P( Z<3) - P( Z <2)
= 0.5 + A(3) - ( 0.5 + A(2))
= A(3) - A( 2)
= 0.49865 - 0.4772 ( From Normal table)
= 0.02145
P( 73 < x < 84 ) = 0.02145
The approximate percentage of cars that remain in service between 73 and 84 months
P( 73 < x < 84 ) = 0.02145 = 2.1 %