Question

TX is the perpendicular bisector of Su. What is the length of SU?

Answer 1

`SU = 20`

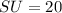
Explanation:

Given

Triangle TSU

Bisector TX

Required

Length of SU

A line is said to be a perpendicular bisector if and only if it divides a line segment into two equal lengths;

This means that line TX divides line SU into two equal part.

This implies that

`SU = SX + UX`

and

`SX = UX`

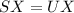
Substitute
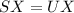`SX = UX`; The expression becomes

`SU = UX + UX`

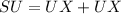
Recall that
`UX = 10`;

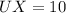So, the above expression becomes

`SU = 10 + 10`

`SU = 20`

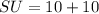
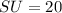
Hence, the length of is 20