Question

Five hundred draws are made at random from the box: True or false? (a) The expected value for the percentage of 1’s among the draws is exactly 25%. (b) The expected value for the percentage of 1’s among the draws is around 25%, give or take 2% or so. (c) The percentage of 1’s among the draws will be around 25%, give or take 2% or so. (d) The percentage of 1’s among the draws will be exactly 25%. (e) The percentage of 1’s in the box is exactly 25%. (f) The percentage of 1’s in the box is around 25%, give or take 2% or so.

Answer 1

Five hundred draws are made at random from the box:

`[60,000 \ |0|'s \ \ \ 20,000 \ |1|'s]`

True or false?

(a) The expected value for the percentage of 1’s among the draws is exactly 25%.

(b) The expected value for the percentage of 1’s among the draws is around 25%, give or take 2% or so.

(c) The percentage of 1’s among the draws will be around 25%, give or take 2% or so.

(d) The percentage of 1’s among the draws will be exactly 25%.

(e) The percentage of 1’s in the box is exactly 25%.

(f) The percentage of 1’s in the box is around 25%, give or take 2% or so.

Answer:

(a) True

(b) False

(c) True

(d) False

(e) True

(f) False

Explanation:

From (a);

We can infer that the expected value for the percentage of 1’s among the draws is exactly 25% is true.

Because ; average of the box =
`(20000)/(60000+20000)`

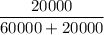
average of the box =
`(20000)/(80000)`

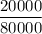
average of the box = 0.25

Thus; the expected value is said to be ; 25%

(b) The expected value for the percentage of 1’s among the draws is around 25%, give or take 2% or so.

Well!, this is false.

Because the expected value for the percentage of 1's among the draws is 25% ,as a result of that there won't be any give and take due to the fact that the expected value involves no probability error.

(c) The percentage of 1’s among the draws will be around 25%, give or take 2% or so.

This is true.

If we determine the standard deviation of the box ; we have :

`SD =\sqrt{(20000)/(80000)*(60000)/(80000)} \\ \\ SD= 0.433`

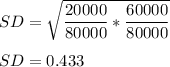
The standard error of the sum can now be calculated as :

= 0.433 ×√500

= 9.682

The standard error of the percentage of 1's is:

`=(9.682)/(500)*100`

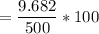
= 1.9364%

≅ 2%

(d) The percentage of 1’s among the draws will be exactly 25%

It is clear and obvious that this is false because if we look at what we have in (c) from above ; we will realize that the percentage of 1's among the draws will be around 25% and not exactly 25%

(e) The percentage of 1’s in the box is exactly 25%.

Yes; this is true. The percentage of 1’s in the box is exactly 25%.

(f) The percentage of 1’s in the box is around 25%, give or take 2% or so.

No; this is false. The percentage of 1’s in the box is exactly 25% and not around 25%, give or take 2% or so.