Question

In a game of rolling a die. If the number showing is even, you win $3, if the number showing is 1 you win $3 and if the number showing is either 3 or 5 you win nothing. Let x be the amount you win,

i. list the probability distribution of x.

ii. What is the amount of dollar you expect to win?

Answer 1

(a)

`\left|\begin{array}c---&---&---\\x&\$0&\$3\\---&---&---\\P(x)&\frac13&\frac23\\---&---&---\\\end{array}\right|`

(b)$2

Explanation:

In the given game of rolling a die. these are the possible winnings.

• If the number showing is even(2, 4, or 6) or 1, you win $3.
• If the number showing is either 3 or 5 you win $0.

There are 6 sides in the die.

`P($obtaining a 1,2,4 or 6)=\frac46=\frac23\\P($obtaining a 3 or 5)=\frac26=\frac13`

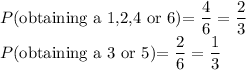
(i)The probability distribution of x.

Let x be the amount won

Therefore:

Probability distribution of x.

`\left|\begin{array}c---&---&---\\x&\$0&\$3\\---&---&---\\P(x)&\frac13&\frac23\\---&---&---\\\end{array}\right|`

(ii) Expected amount of dollar won

Expected Amount

`=\sum x_iP(x_i)\\=(0*\frac13)+(3*\frac23)\\=\$2\\`

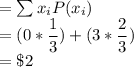
You would expect to win $2.