Question

A science test, which is worth 100 points, consists of 24 questions. Each question is worth either 3 points or 5 points. If x is the number of 3-point questions and y is the number of 5-point questions, the system shown represents this situation. x + y = 24 3x + 5y = 100 What does the solution of this system indicate about the questions on the test? The test contains 4 three-point questions and 20 five-point questions. The test contains 10 three-point questions and 14 five-point questions. The test contains 14 three-point questions and 10 five-point questions. The test contains 20 three-point questions and 8 five-point questions.

Answer 1

The solution of this system is (10,14) and it means that there are 10 three point questions and 14 five point questions.

Explanation:

In order to find the number of questions of each kind we need to solve the given system as shown below:

`\left \{ {{x+y=24} \atop {3x + 5y=100}} \right.`

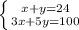
If we multiply the first equation by -3 and sum it with the second equation we can isolate the "y" variable and solve for its value:

`\left \{ {{-3x -3y=-72} \atop {3x + 5y=100}} \right.\\ \\-3y + 5y = -72 + 100\\2y = 28\\y = 14`

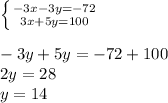
We can use this value to find "x":

`x + y = 24\\x + 14 = 24\\x = 24 - 14\\x = 10`

The solution of this system is (10,14) and it means that there are 10 three point questions and 14 five point questions.