The heights of a certain type of tree are approximately normally distributed with a mean height p = 5 ft and a standard deviation o = 0.4 it. Which statement must be true?

Question

asked Oct 17, 2021 95.3k views

2 Answers

Uchenna Nwanyanwu · Answer 1 · 2021-10-22T05:42:18+0000

Answer:

A tree with a height of 6.2 ft is 3 standard deviations above the mean

Explanation:

⇒
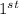1^s^t statement: A tree with a height of 5.4 ft is 1 standard deviation below the mean(FALSE)

an X value is found Z standard deviations from the mean mu if:

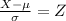
$(X-\mu)/(\sigma) = Z$

In this case we have:
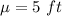
$\mu=5\ ft$
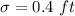
$\sigma=0.4\ ft$

We have four different values of X and we must calculate the Z-score for each

For X =5.4\ ft

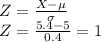
$Z=(X-\mu)/(\sigma)\\Z=(5.4-5)/(0.4)=1$

Therefore, A tree with a height of 5.4 ft is 1 standard deviation above the mean.

⇒
2^n^d statement:A tree with a height of 4.6 ft is 1 standard deviation above the mean. (FALSE)

For X =4.6 ft

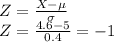
$Z=(X-\mu)/(\sigma)\\Z=(4.6-5)/(0.4)=-1$

Therefore, a tree with a height of 4.6 ft is 1 standard deviation below the mean .

⇒
3^r^d statement:A tree with a height of 5.8 ft is 2.5 standard deviations above the mean (FALSE)

For X =5.8 ft

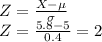
$Z=(X-\mu)/(\sigma)\\Z=(5.8-5)/(0.4)=2$

Therefore, a tree with a height of 5.8 ft is 2 standard deviation above the mean.

⇒
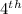4^t^h statement:A tree with a height of 6.2 ft is 3 standard deviations above the mean. (TRUE)

For X =6.2\ ft

$Z=(X-\mu)/(\sigma)\\Z=(6.2-5)/(0.4)=3$

Therefore, a tree with a height of 6.2 ft is 3 standard deviations above the mean.