Answer:
B) 16%
Explanation:
First, we need to standardize 58 and 66 inches using the following equation:
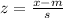
Where m is the mean and s is the standard deviation, so 58 and 66 are equivalent to:

Then, the percent of men whose heights falls between 58 inches and 66 inches is calculated as:
P(58<z<66) = P(-3<z<-1)
So, using the normal table, we get:
P(-3<z<-1) = P(z<-1) - P(z<-3)
P(-3<z<-1) = 0.1587 - 0.0013
P(-3<z<-1) = 0.1574
Finally, 0.1574 rounded to the nearest whole percent is equal to 16%