Question

Which expression represents the volume, in cubic units, of

the composite figure?
pi(5^2)(13)-1/3pi(5^2)(12) pi(5^2)(13)-1/3pi(5^2)(25)
pi(5^2)(13)+1/3pi(5^2)(12)
pi(5^2)(13+1/3pi(5^2)(25)

Answer 1

C

Explanation:

Answer 2

C.

`V = \pi * 5^2(13) +(1)/(3)\pi * 5^2 (12)`

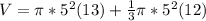
Explanation:

Given:

The figure is a combination of a cone and a cylinder

Radius (of both),
`r = 5`

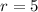
Height of Cylinder,
`H_1 = 13`

Height of Cone,
`H_2 = 25 - 13 = 12`

Required:

Volume of the figure

Let V represents the volume of the figure;

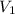`V_1` represents the volume of the cylinder

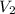`V_2` represents the volume of the cone

So,
`V = V_1 + V_2`

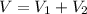
Where
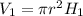`V_1 = \pi r^2H_1` and
`V_2 = (1)/(3)\pi r^2 H_2`

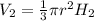
So, V becomes

`V = \pi r^2H_1 + (1)/(3)\pi r^2 H_2`

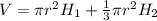
Factorize the above expression

`V = \pi r^2(H_1 + (1)/(3)H_2)`

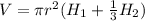
Substitute
`r = 5`;
`H_1 = 13`;
`H_2 = 12`

`V = \pi * 5^2(13 + (1)/(3) * 12)`

`V = \pi * 5^2(13 + (1)/(3) * 12)`

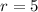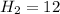
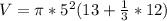
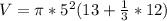
Open Bracket

`V = \pi * 5^2(13) +\pi * 5^2 (1)/(3) (12)`

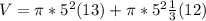
Reorder

`V = \pi * 5^2(13) +(1)/(3)\pi * 5^2 (12)`

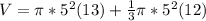
From the list of options given, option c is correct.