Question

Crash testing evaluates the ability of an automobile to withstand a serious accident. A simple random sample of 12 small cars were subjected to a head-on collision at 40 miles per hour. Of them, 8 were totaled, meaning that the cost of repairs is greater than the value of the car. Another sample of 15 large cars were subjected to the same test, and 5 of them were totaled. Find a 95% confidence interval for the difference in the proportions of small cars and large cars that were totaled.

Answer 1

The 95% confidence interval for the difference between proportions is (-0.046, 0.713).

Explanation:

We want to calculate the bounds of a 95% confidence interval.

For a 95% CI, the critical value for z is z=1.96.

The sample 1 (small cars), of size n1=12 has a proportion of p1=0.6667.

`p_1=X_1/n_1=8/12=0.6667`

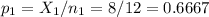
The sample 2, of size n2=15 has a proportion of p2=0.3333.

`p_2=X_2/n_2=5/15=0.3333`

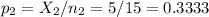
The difference between proportions is (p1-p2)=0.3333.

`p_d=p_1-p_2=0.6667-0.3333=0.3333`

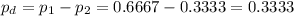
The pooled proportion, needed to calculate the standard error, is:

`p=(X_1+X_2)/(n_1+n_2)=(8+5)/(12+15)=(13)/(27)=0.4815`

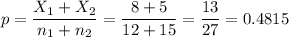
The estimated standard error of the difference between means is computed using the formula:

`s_(p1-p2)=\sqrt{(p(1-p))/(n_1)+(p(1-p))/(n_2)}=\sqrt{(0.4815*0.5185)/(12)+(0.4815*0.5185)/(15)}\\\\\\s_(p1-p2)=√(0.0208+0.0166)=√(0.0374)=0.1935`

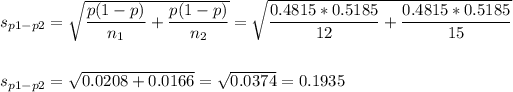
Then, the margin of error is:

`MOE=z \cdot s_(p1-p2)=1.96\cdot 0.1935=0.3793`

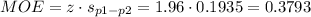
Then, the lower and upper bounds of the confidence interval are:

`LL=(p_1-p_2)-z\cdot s_(p1-p2) = 0.3333-0.3793=-0.046\\\\UL=(p_1-p_2)+z\cdot s_(p1-p2)= 0.3333+0.3793=0.713`

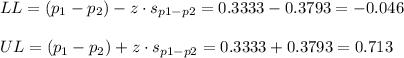
The 95% confidence interval for the difference between proportions is (-0.046, 0.713).