Answer:
Due to the higher Z-score, Norma should be offered the job
Explanation:
Z-score:
In a set with mean
 and standard deviation
and standard deviation
 , the zscore of a measure X is given by:
, the zscore of a measure X is given by:

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.
In this question:
Whoever has the higher z-score should be offered the job.
Alissa:

So

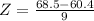
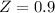
Morgan:
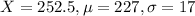
So

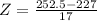

Norma:
![X = 7.96, \mu = 6.7, \sigma = 0.7[tex]</p><p>So</p><p>[tex]Z = (X - \mu)/(\sigma)](https://img.qammunity.org/2021/formulas/mathematics/college/u9m1117pagp1he2vo2tj9gyeqh8oieg9er.png)
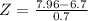
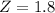
Due to the higher Z-score, Norma should be offered the job