Question

Given the line 2x - 3y - 5 = 0, find the slope of a line that is perpendicular to this line. ​

Answer 1

Given ↓

• The equation of the line 2x - 3y -5 = 0

To find ↓

• The slope of the line perpendicular to this line

Calculations ↓

First of all, we need to add 5 on both sides :

2x-3y=5

Now, subtract 2x on both sides ↓

-3y=5-2x

Or

-3y=-2x+5

Now we need to divide by -3 on both sides to get y all by itself ↓

y=-2/-3+5/-3

`\boxed{\\\begin{minipage}{2cm}y=$\displaystyle(2)/(3) x-(5)/(3)$ \\ \end{minipage}}`

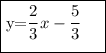
Now determining the slope is facile.

If lines are perpendicular to each other, they

1. Intersect at a 90º angle
2. Have slopes that are opposite reciprocals

In order to find the opposite reciprocal of a fraction, we need to switch the numerator & the denominator places and then change the fraction's sign as follows ↓

(slope = 2/3)

Switch the numerator and denominator places ↓

3/2

Change the fraction's sign ↓

-3/2

Therefore the slope's -3/2

hope helpful ~

Answer 2

The slope of the line would be -3/2.

Explanation:

Let's get this equation into the proper slope form:

2x - 3y - 5 = 0

2x - 3y + 3y - 5 = 0 + 3y

2x - 5 = 3y

(1/3)(2x - 5) = 3y * 1/3

2/3x - 5/3 = y

Since the slope is 2/3, we need to find the negative reciprocal of that number, which is -3/2.