Question

Consider the enlargement of the rectangle. A smaller rectangle with length of x inches and width of three-fifths inch. A larger rectangle with length of 20 inches and width of 12 inches. What is the correct multiplication of a cross product to solve for the missing dimension?

Answer 1

Step-byConsider the enlargement of the rectangle.

A smaller rectangle with length of x inches and width of three-fifths inch. A larger rectangle with length of 20 inches and width of 12 inches.

Use the proportion to find the missing dimension of the original rectangle.

1. Set up the proportion: StartFraction x over three-fifths EndFraction = StartFraction 20 over 12 EndFraction

2. Use cross products: 12(x) = 20(3

5

)

3. Simplify:

12x =

⇒ 12

4. Divide:

x =

1

-step explanation:

so the REAL answer is first 12 then 1

Answer 2

The new rectangle is 20 times larger than the original rectangle.

So multiply both length and width of smaller rectangle with 20 to get larger rectangle

x=1

Explanation:

Lenght of smalled rectangle = x

Width of smaller rectangle = 3/5 inches

Lenght of larger rectangle = 20

Width of larger rectangle = 12

Find the ratio of width of large rectangle to small rectangle:
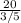`(20)/(3/5)` : 20

Divide length of larger rectangle with 20 to find x:
`(20)/(20)=1`