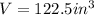Question

Find the volume of a right circular cone that has a height of 8.1 in and a base with a diameter of 7.6 in. Round your answer to the nearest tenth of cubic inch

Answer 1

`V=122.5in^3`

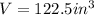
Explanation:

The volume of a right circular cone is given by:

`V=(\pi r^2h)/(3)`

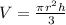
where r is the radius of the circle and h is the height of the cone, and
`\pi` is a constant
`\pi=3.1416`.

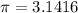According to the problem the height is:

`h=8.1 in`

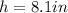
and we don't have the radius but we have the diameter, which is useful to find it. We just divide the diameter by 2 to find the radius:

`r=(d)/(2)=(7.6in)/(3)=3.8in`

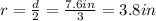
Now, we can find the volume by substituting all the known values:

`V=(\pi r^2h)/(3)`

`V=((3.1416)(3.8in)^2(8.1in))/(3) \\\\V=((3.1416)(14.44in^2)(8.1in))/(3) \\\\V=(367.454in^3)/(3) \\\\V=122.485`

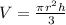
Rounding the volume to the nearest tenth of cubic inch we get:

`V=122.5in^3`