The images to solve this problem is in the attachment.
Answer:
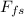 = 671.0 N;
= 671.0 N;
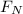 = 300 N
= 300 N
Explanation: From the image in the attachment and knowing that the box is in equilibrium, i.e., the "sum" of all the forces is 0, it is possible to conclude that:
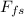 =
=
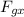 and
and
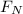 =
=
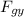
Using trigonometry, shown in the second attachment, the values for each force are:
sin 20° =
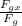
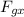 =
=
 . sin(20)
. sin(20)
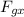 = 735.0.913
= 735.0.913
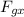 = 671.0
= 671.0
cos 20° =
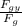
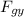 =
=
 . cos (20)
. cos (20)
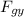 = 735.0.408
= 735.0.408
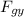 = 300
= 300
The force of static friction is 671N and normal force is 300N