Answer:
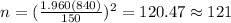
So the answer for this case would be n=12 rounded up to the next integer
Step-by-step explanation:
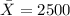 represent the sample mean
represent the sample mean
 population mean (variable of interest)
population mean (variable of interest)
s=840 represent the sample standard deviation
n represent the sample size
The margin of error is given by this formula:
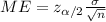 (a)
(a)
And on this case we have that ME =150 and we are interested in order to find the value of n, if we solve n from equation (a) we got:
 (b)
(b)
The critical value for 95% of confidence interval, the significance level if 5% and the critical value would be
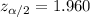 , replacing into formula (b) we got:
, replacing into formula (b) we got:
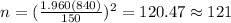
So the answer for this case would be n=12 rounded up to the next integer