Question

A tank with a constant volume of 3.72 m3 contains 22.1 moles of a monatomic ideal gas. The gas is initially at a temperature of 300 K. An electric heater is used to transfer 4.5 × 104 J of energy into the gas. It may help you to recall that CV = 12.47 J/K/mole for a monatomic ideal gas, and that the number of gas molecules is equal to Avagadros number (6.022 × 1023) times the number of moles of the gas.

a) What is the temperature of the gas after the energy is added?___K

b) What is the change in pressure of the gas?____Pa

c) How much work was done by the gas during this process?____J

Answer 1

a) 463.29 K

b) 8065.65 Pa

c) 0 J

Step-by-step explanation:

The parameters given are;

Volume of the tank, V = 3.72 m³

Number of moles of gas present in the tank, n = 22.1 moles

Temperature of the gas before heating, T₁ = 300 k

Heat added to the gas, ΔQ = 4.5 × 10⁴ J

Specific heat capacity at constant volume,
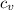`c_v`, for monatomic gas = 12.47 J/K/mole

Avogadro's number = 6.022 × 10²³ particles per mole

a) ΔQ = n ×
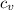`c_v` × ΔT

Where:

ΔT = T₂ - T₁

T₂ = Final temperature of the gas

Hence, by plugging in the values, we have;

4.5 × 10⁴ = 22.1 × 12.47 × (T₂ - 300)

`T_(2) - 300 = (4.5* 10^(4))/(22.1* 12.47)`

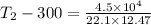
T₂ = 300 + 163.29 = 463.29 K

b) The pressure of the gas is found from the relation;

P×V = n×R×T

`P = (n * R * T)/(V)`

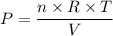
Where:

P = Pressure of the gas

R = Universal gas constant = 8.3145 J/(mol·K)

T = Temperature of the gas

V = Volume of the gas = 3.72 ³ (constant)

n = Number of moles of gas present = 22.1 moles (constant)

Hence the change in pressure is given by the relation;

`\Delta P = (n * R * (T_2 - T_1))/(V) = (n * R * \Delta T)/(V)`

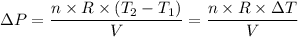
Plugging in the values, we have;

`\Delta P = (22.1 * 8.3145 * 163.29)/(3.72) = 8065.65 \, Pa`

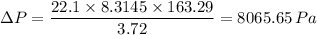
c) Work done, W, by the gas is given by the area under the pressure to volume graph which gives;

W = f(P) × ΔV

The volume given in the question is constant

∴ ΔV = 0

Hence, W = f(P) × 0 = 0 J

No work done by the gas during the process.