Hey ! there
Answer:
Explanation:
In this question we are given with an equation that is 8 = -x + 10 and we have also given its solution that is 2 .And We are asked to tell whether the solution of given equation is true or false .
For finding that we must have to solve the equation .
Solution : -
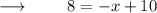
Step 1 : Flipping the equation so that there's ease in solving :
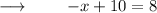
Step 2 : Subtracting with 10 on both sides :
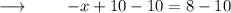
On further calculations , We get :

Step 3 : Multiplying with -1 on both sides :
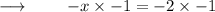
We get :
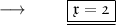
- Therefore , it's TRUE that 2 is the solution of given equation .
Alternative Solution : -
There's another easy way to check whether 2 is solution of given equation or not . This is done by substituting value of x that is 2 in given equation that is 8 = -x + 10 . So substituting value of x ( solution ) in given equation :
As we can see that left side is equal to right side .
- Therefore , it's TRUE that 2 is the solution of given equation .
#Keep Learning