Question

According to the Rational Root Theorem, Negative two-fifths is a potential rational root of which function?

f(x) = 4x4 – 7x2 + x + 25
f(x) = 9x4 – 7x2 + x + 10
f(x) = 10x4 – 7x2 + x + 9
f(x) = 25x4 – 7x2 + x + 4

Answer 1

D. f(x) = 25x^4 - 7x^2 + x + 4.

Explanation:

The correct answer to your question is D.

Answer 2

Neither expression satisfies the given rational root.

Explanation:

To find the right answer, we just need to replace the given root in each expression and see which one gives zero.

First expression.

`f(x)=4x^(4) -7x^(2) +x+25\\f(-(2)/(5))= 4(-(2)/(5))^(4) -7(-(2)/(5))^(2) +(-(2)/(5))+25=(64)/(625)-(28)/(25) -(2)/(5) +25 \approx 23.58`

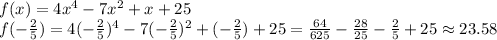
Second expression.

`f(x)=9x^(4)-7x^(2) +x+10=9(-(2)/(5) )^(4) -7(-(2)/(5) )^(2) +(2)/(5) +10 \approx 9.5`

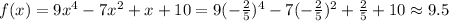
Third expression.

`f(x)=10x^(4)-7x^(2) +x+9=10(-(2)/(5) )^(4) -7(-(2)/(5))^(2) +(-(2)/(5))+9 \approx 7.7`

Fourth expression.

`f(x)=25x^(4)-7x^(2) +x+4=25(-(2)/(5) )^(4) -7(-(2)/(5))^(2) +(-(2)/(5))+4 \approx 3.12`

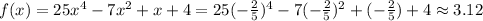
Therefore, neither expression satisfies the given rational root.