Answer:
171.5Hz,514.5Hz and 857.5Hz
Step-by-step explanation:
We are given that
Distance between two loudspeaker,d=2.65 m
Distance of listener from one end=19.1 m
Distance of listener from other end=20.1 m
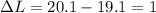 m
m
Speed of sound,v=343m/s
For destructive interference
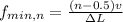
Using the formula and substitute n=1
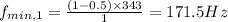
For n=2

For n=3
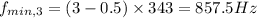
Hence, the three lowest frequencies that give minimum signal (destructive interference) at the listener's location is given by
171.5Hz,514.5Hz and 857.5Hz