Answer:
No real roots or no real zeros.
Explanation:
The given function is
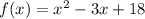
We need to find the number of real zeroes.
The given function is a quadratic function.
Let a quadratic function is
 .
.
If
 , then the function has no real root.
, then the function has no real root.
If
 , then the function has one real root with multiplicity 2.
, then the function has one real root with multiplicity 2.
If
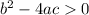 , then the function has two real roots.
, then the function has two real roots.
In the given function,

So,
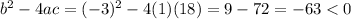
The value of discriminant is -63.
Therefore, the given function has no real root or no real zeros.