Question

Professor Jennings claims that only 35% of the students at Flora College work while attending school. Dean Renata thinks that the professor has underestimated the number of students with part-time or full-time jobs. A random sample of 78 students shows that 36 have jobs. Do the data indicate that more than 35% of the students have jobs

Answer 1

`z=\frac{0.462 -0.35}{\sqrt{(0.35(1-0.35))/(78)}}=2.074`

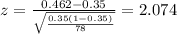
Now we can calculate the p value with this probability:

`p_v =P(z>2.074)=0.019`

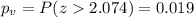
If we use a significance level os 0.05 we see that the p value is lower than the significance level so then we can conclude that the true proportion of students with jobs is higher than 0.35 for this case. If we decrease the significance level to 1% the result changes otherwise not.

Explanation:

Information given

n=78 represent the random sample taken

X=36 represent the students with jobs

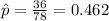`\hat p=(36)/(78)=0.462` estimated proportion of students with jobs

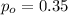`p_o=0.35` is the value that we want to test

z would represent the statistic

`p_v` represent the p value

Hypothesis to test

We want to test if the proportion of students with jobs is higher than 0.35, the system of hypothesis are:

Null hypothesis:
`p \leq 0.35`

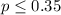
Alternative hypothesis:
`p > 0.35`

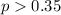
The statistic is given by:

`z=\frac{\hat p -p_o}{\sqrt{(p_o (1-p_o))/(n)}}` (1)

Replacing the info we got:

`z=\frac{0.462 -0.35}{\sqrt{(0.35(1-0.35))/(78)}}=2.074`

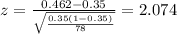
Now we can calculate the p value with this probability:

`p_v =P(z>2.074)=0.019`

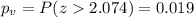
If we use a significance level os 0.05 we see that the p value is lower than the significance level so then we can conclude that the true proportion of students with jobs is higher than 0.35 for this case. If we decrease the significance level to 1% the result changes otherwise not.