Answer:
The probability that at least 8 of the vest worn will be black is 0.75490
Explanation:
The parameters given are;
The percentage of black vest worn = 70%, p₀ = 0.7
Number of men in sample, n = 10
Required number of men who wore black = 8
Proportion of sample
 = 8/10 = 0.8
= 8/10 = 0.8
The z score of a proportion is given by the relation;
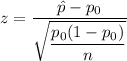
Plugging in the vales, we have;
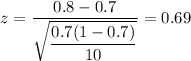
From the z table we have probability = p(z < 0.69) = 0.75490
The probability that at least 8 of the vest worn will be black = 0.75490.