Question

A sample mean, sample size, and population standard deviation are given. Use the one-mean z-test to perform the required hypothesis test at the given significance level. Use the critical -value approach.

= 20.5, n = 11 , σ = 7, H0: μ = 18.7; Ha: μ ≠ 18.7, α = 0.01

Answer 1

Answer:Answer:

B. 18.7 ± 9.7

Explanation:

Answer 2

Z = 0.8528 < 2.576

The calculated value Z = 0.8528 < 2.576 at 0.01 level of significance

Null hypothesis is Accepted at 0.01 level of significance.

There is no significance difference between the means

Step-by-step explanation:

Given data

size of the sample 'n' = 11

mean of the sample x⁻ =20.5

Mean of the Population μ = 18.7

Standard deviation of Population σ = 7

Test statistic

`Z = (x^(-) -mean)/((S.D)/(√(n) ) )`

`Z = (20.5 -18.7)/((7)/(√(11) ) )`

`Z = (1.8)/(2.1105)`

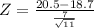
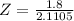
Z = 0.8528

critical Value

`Z_{(\alpha )/(2) } = Z_{(0.01)/(2) } = Z_(0.005) = 2.576`

The calculated value Z = 0.8528 < 2.576 at 0.01 level of significance

Null hypothesis is Accepted at 0.01 level of significance.

There is no significance difference between the means