Answer:
There is sufficient statistical evidence to prove that the standard deviation of the technology-related workers and the standard deviation of the non-technology workers are equal.
Explanation:
Here we have our null hypothesis as H₀: σ² = s²
Our alternative hypothesis is then Hₐ: σ² ≠ s²
We therefore have a two tailed test
To test the hypothesis of difference in standard deviation which is the Chi squared test given as follows
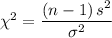
Where:
n = Size of sample
s² = Variance of sample = 950²
σ² = Variance of population = 650²
Degrees of freedom = n - 1 = 71 - 1 = 70
α = Significance level = 0.1
Therefore, we use 1 - 0.1 = 0.9
From the Chi-square table, we have the critical value as
1 - α/2 = 51.739,
α/2 = 90.531
Plugging the values in the above Chi squared test equation, we have;
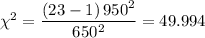
Therefore, since the test value within the critical region, we do not reject the null hypothesis, hence there is sufficient statistical evidence to prove that the standard deviation of the technology-related workers and the standard deviation of the non-technology workers are equal.