Question

Segment AB falls on line 2x − 4y = 8. Segment CD falls on line 4x + 2y = 8. What is true about segments AB and CD?

They are perpendicular because they have the same slope of −2.

They are perpendicular because they have slopes that are opposite reciprocals of −2 and .

They are lines that lie exactly on top of one another because they have the same slope and the same y-intercept.

They are lines that lie exactly on top of one another because they have the same slope and a different y-intercept.

Answer 1

They are perpendicular because they have slopes that are opposite reciprocals of −2 and .

Step-by-step explanation:

I took the test and the dot is 1/2

Answer 2

They are perpendicular because they have slopes that are opposite reciprocals of −2 and .

Explanation:

The given lines are

`2x-4y=8`

`4x+2y=8`

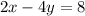
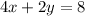
Let's rewrite each equation in the form
`y=mx+b`

`2x-4y=8\\2x-8=+4y\\y=(2x-8)/(4) \\y=(x)/(2)-2`

`4x+2y=8\\2y=8-4x\\y=(8-4x)/(2)\\ y=4-2x`

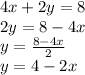
Now, let's use the perpendicular rule

`m_(1) * m_(2) =-1\\(1)/(2) * (-2)=-1 \implies -1=-1`

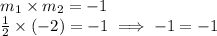
As you can observe, the slopes satisfy the perpendicular rule, that means the lines are perpendicular.

Therefore, the right answer is the second choice because it states the rule of perpendicularity between the given lines.