Question

Sara is a drummer in her school is marching band she wants to make a geometrically similar model of a snare drum for her stuffed animals. To find the dimensions she should use to make a cylindrical model with a scale of 1 : 4, Sara measures the radius and height of a snare drum and multiplies each dimension by 1/2. Which statement is true?

A. A cylinder with Sara’s dimensions will be geometrically similar, and the scale factor will be 1:4


B. A cylinder with Sara’s dimensions will be geometrically similar, but the scale factor will be 1:2


C. A cylinder with Sara’s dimensions will be geometrically similar, but the scale factor will be 1:8


D. A cylinder with Sara’s dimensions will not be geometrically similar

Answer 1

Option B.

Explanation:

Let the radius of the snare drum = r

and radius of the model = R

Ratio of the dimensions of the snare drum and the model = 1 : 4

So,
`(r)/(R)=(1)/(4)`

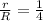
Now as per question, dimensions of the snare drum is multiplied by a scale factor of
`(1)/(2)`

Radius of the snare drum =
`(r)/(2)`

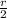
Ratio of the radius of the snare drum and cylindrical model ,

`((r)/(2))/(R) =(1)/(4)`

`(r)/(2R)=(1)/(4)`

`(r)/(R)=(1)/(2)`

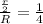
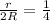
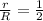
Therefore, the cylinder with Sara's dimensions will be geometrically similar but the scale factor will be 1 : 2

Option B is the answer.