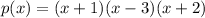Answer:
Using synthetic division suffices to answer your question:
Explanation:
Synthetic division is the process by one reduces a large polynomial in your case
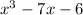 by a binomial in your case
by a binomial in your case
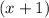 .
.
To do so one does the following:

Since we divided by a linear binomial it reduces the power by one which produces the following quadratic:

Which can be factored in the following, and I will provide the complete factorization as well: