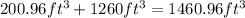Question

Find the volume of the composite figure round to the nearest tenth

Answer 1

Answer:
`1460.96ft^3`

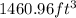
Explanation:

You have a cylinder and a cuboid. Find each of these figures' volume individually and add them together.

Cylinder:

height = 4ft

radius = 4ft

`Formula: V=\pi r^2h`

`V=(3.14)(4ft)^2(4ft)\\V=(3.14)(16ft^2)(4ft)\\V=(3.14)(64ft^3)\\V=200.96ft^3`

---------------------------------------------------------------------------------------------------

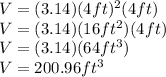
Cuboid:

`Formula: V = l*w*h`

`V=(15ft)(7ft)(12ft)\\V=1260ft^3`

------------------------------------------------------------------------------------------------------

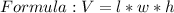
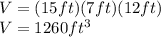
Add these two volumes together.

`200.96ft^3+1260ft^3=1460.96ft^3`