Question

A pizza shop sells pizza by the slice. They must make two different sizes of pizza - a 12-inch pizza which they cut into 60-degree slices and a 14-inch pizza which they cut into 45-degree slices. They sell a slice of each one for the same price. Which is the better deal? Justify your answer, by showing your work.

Answer 1

14 inch pizza.

Explanation:

A pizza is the shape of a circle which is 360 degrees.

For the 12 in pizza, it is being divided into 60 degree slices.

360/60= 6 slices.

The area of the pizza is calculates with
`\pi *r^2` so we must find the radius of the pizza, r. 12 inch is the diameter which is 2r so half of the diameter= r.

12/2=6=r

So the area of the 12 inch pizza is = pi*6*6= 36
`\pi`

36
`\pi`/6 for 6 slices means each slice is 6 pi in area.

For the 14 inch pizza, it is being divided into 45 degree slices.

360/45= 8

The radius = d/2= 14/2= 7

The area =
`\pi`*7*7 = 49pi

For each slice 49
`\pi`/8= 6.125
`\pi`

Since 6.125
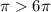`\pi > 6\pi` that means that the 14 inch pizza slice is a better deal because you get more food for the same amount of money.

Answer 2

14inch pizza

Explanation:

1. Compare the area of both slices

For 12in, A = (pi(6^2))/6 = 36pi/6 = 18.9

For 14in, A = (pi(7^2))/7 = 49pi/8 = 19.2