Answer:
1. The error in the assumption is taking the sample mean,
 , as being equal to the population mean, μ
, as being equal to the population mean, μ
2. To correct the error, the manager will need to generate the confidence interval based on the population standard deviation on the acceptable values of the mean using the following relation;
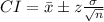
Explanation:
We note that from the central limit theorem as the size of the sample, n, becomes more and more larger, the mean of the sample,
 , approaches that of the population mean, μ, therefore as the grocery store manager uses the sample mean as the population mean's point estimate an error will be observed based on the size of the sample compared to the population, where the difference between the two means (the population mean and the sample mean) is
, approaches that of the population mean, μ, therefore as the grocery store manager uses the sample mean as the population mean's point estimate an error will be observed based on the size of the sample compared to the population, where the difference between the two means (the population mean and the sample mean) is
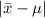
1. The error in the assumption is taking the sample mean,
 , as being equal to the population mean, μ
, as being equal to the population mean, μ
2. To correct the error, the manager will need to generate the confidence interval based on the population standard deviation on the acceptable values of the mean using the following relation;
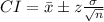
Where:
σ = Population standard deviation = $30
z = z value at 95% confidence level = 1.96
 = Sample mean $160
= Sample mean $160
n = Sample size = 10
Plugging in the values, we have;
$141.4 <
 < $178.6
< $178.6
Hence the expected value of the mean should be between $141.4 and $178.6.