Question

Secant TP and tangent TR intersect at point 7. Chord SR and chord PQ intersect

at point V. Find the values of x and y. If necessary, round to the nearest tenth.

AN

x = 11.6

y = 11.6

= 11.6

y= 23.2

x = 18.3

y = 36.6

Answer 1

(C)x=11.6, y=23.2

Explanation:

Using Theorem of Intersecting Secant and Tangent

Applying this theorem in the diagram, we have:

`TQ$ X TP=TR^2`

`10(10+x+4)=16^2\\10(14+x)=256\\140+10x=256\\10x=256-140\\10x=116\\$Divide both sides by 10\\x=11.6`

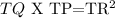
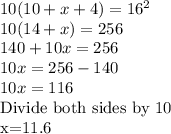
Next, we apply Theorem of Intersecting Chords

PV X VQ=SV X VR

4 X x= 2 X y

Recall earlier we got: x=11.6

2y=4 X 11.6

2y=46.4

Divide both sides by 2

y=46.4/2=23.2

Therefore: x=11.6, y=23.2