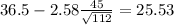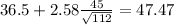Answer:
a)

b) For this case we have 95% of confidence that the true mean would be between
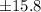 units respect the true mean.
units respect the true mean.
c)
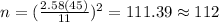
So the answer for this case would be n=112
d)
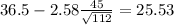
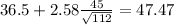
Explanation:
Part a
For this case we know that the cinfidence interval for the true mean is given by:
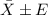
Where E represent the margin of error. For this case we have the confidence interval at 95% of confidence and we can estimate the margin of error like this:

Part b
For this case we have 95% of confidence that the true mean would be between
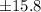 units respect the true mean.
units respect the true mean.
Part c
The margin of error is given by this formula:
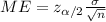 (a)
(a)
And on this case we have that ME =11 and we are interested in order to find the value of n, if we solve n from equation (a) we got:
 (b)
(b)
The critical value for 99% of confidence interval now can be founded using the normal distribution. The critical value would be
 , replacing into formula (b) we got:
, replacing into formula (b) we got:
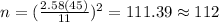
So the answer for this case would be n=112
Part d
The confidence interval for the mean is given by the following formula:
 (1)
(1)
Replcaing the info given we got: