Question

The position of a ball after it is kicked can be determined by using the function f left parenthesis x right parenthesis equals negative 0.11 x squared plus 2.2 x plus 1f(x)=−0.11x2+2.2x+1​, where​ f(x) is the​ height, in​ feet, above the ground and x is the horizontal​ distance, in​ feet, of the ball from the point at which it was kicked. What is the height of the ball when it is​ kicked? What is the highest point of the ball in the​ air?

Answer 1

When kicked, the height of the ball is 1 feet. The highest point for the ball's trajectory is 12 feet.

Explanation:

We are given that the height is given by
`f(x)=-0.11x^2+2.2x+1`. The distance between the ball to the point where it was kicked is 0 right at the moment the ball was kicked. So, the height of the ball, when it was kicked is f(0) = -0.11*0 + 2.2*0 +1 = 1.

To determine the highest point, we will proceed as follows. Given a parabola of the form x^2+bx + c, we can complete the square by adding and substracting the factor b^2/4. So, we get that

`x^2+bx+c = x^2+bx+(b^2)/(4) - (b^2)/(4) +c = (x+(b)/(2))^2+c-(b^2)/(4)`.

In this scenario, the highest/lowest points is

We will complete the square for
`x^2-20x`. In this case b=-20, so

`f(x) = -0.11(x^2-20x+(400)/(4)-(400)/(4))+1 = -0.11(x^2-20x+100-100)+1`

We can distribute -0.11 to the number -100, so we can take it out of the parenthesis, then

`f(x) = -0.11(x^2-20x+100)+1+100*0.11 = -0.11(x^2-20x+100)+1+11 = -0.11(x-10)^2+12`

So, the highest point in the ball's trajectory is 12 feet.

Answer 2

Initial height = 1ft

Heighest height = 12ft

Explanation:

In order to solve this problem, we can start by graphing the given height function. This will help us visualize the problem better and even directly finding the answers, since if you graph it correctly, you can directly find the desired values on the graph. (See attached picture)

So, the initical height happens when the x-value is equal to zero (starting point) so all we need to do there is substitute every x for zero so we get:

`f(x)=-0.11x^(2)+2.2x+1`

`f(0)=-0.11(0)^(2)+2.2(0)+1`

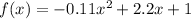
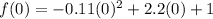
which yields:

`f(0)=1`

so the height of the ball when it is kicked is 1 ft.

In order to find the highest point of the ball in the air, we must determine the x-value where this will happen and that can be found by calculating the vertex of the parabola. (see the graph)

the vertex is found by using the following formula:

`x=-(b)/(2a)`

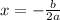
in order to find "a" and "b" we must compare the given function with the standard form of a quadratic function:

`f(x)=ax^(2)+bx+c`

`f(x)=-0.11x^(2)+2.2x+1`

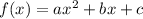
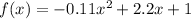
so:

a=-0.11

b=2.2

c=1

so the vertex formula will be:

`x=-(b)/(2a)`

`x=-(2.2)/(2(-0.11))`

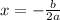
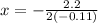
so we get that the highest point will happen when x=10ft

so the highest point will be:

`f(10)=-0.11(10)^(2)+2.2(10)+1`

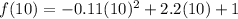
f(10)=12ft

so the highes point of the ball in the air will be (10,12) which means that the highest the ball will get is 12 ft.