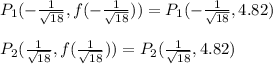Question

Consider the function below. (If an answer does not exist, enter DNE.) f(x) = 1 2 x4 − 4x2 + 5 (a) Find the interval of increase. (Enter your answer using interval notation.) Find the interval of decrease. (Enter your answer using interval notation.) (b) Find the local minimum value(s). (Enter your answers as a comma-separated list.) Find the local maximum value(s). (Enter your answers as a comma-separated list.) (c) Find the inflection points.

Answer 1

Please, read the answer below.

Explanation:

You have the following function:

`f(x)=12x^4-4x^2+5`

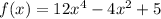
(a) To find the intervals of increase or decrease of f(x) you first calculate the derivative of f(x):

`(df)/(dx)=(d)/(dx)[12x^4-4x^2+5]\\\\(df)/(dx)=12(4)x^3-4(2)x=48x^3-8x` (2)

Next, you equal the derivative to zero and obtain the roots of the obtained polynomial:

`48x^3-8x=0\\\\6x^3-x=0\\\\x(6x^2-1)=0`

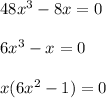
Then, you have the following roots for x:

`x_1=0\\\\x_(2,3)=\pm \sqrt{(1)/(6)}` = ±0.40

Hence, there are three special points.

Next, you evaluate the derivative (expression (2)) for the x values close to the x1, x2 and x3. The values of the derivative give to us the value of the slope of a tangent line in that point, and so, if the function increases or decreases:

First interval, for a number lower than -0.40

`48(-0.41)^3-8(-0.41)=-0.02<0`

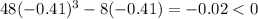
The function decreases in the interval:

`(-\inft,-(1)/(√(6)))`

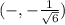
It is necessary that after x=-0.40 the function increases until the next special point, that is x=0. Then, the interval in which the function increases is:

`(-(1)/(√(6)),0)`

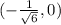
By symmetry, from the point x=0 until x=0.40 the function decreases.

`(0,(1)/(√(6)))`

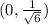
Next, you evaluate the expression (2) for a number higher than 0.40:

`48(0.41)^3-8(0.41)=0.02>0`

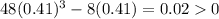
Then, the function increases for the following interval:

`((1)/(√(6)),+\infty)`

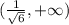
(b) Due to the results obtained in the previous step you can conclude that the local minimum are:

`x_(min)=-(1)/(√(6))\\\\x_(min)=(1)/(√(6))`

`P_1(-(1)/(√(6)),f(-(1)/(√(6))))=P_1(-(1)/(√(6)),4.66)\\\\P_2((1)/(√(6)),f((1)/(√(6))))=P_2((1)/(√(6)),4.66)`

`P_1(-(1)/(√(6)),0)\\\\P_2((1)/(√(6)),0)`

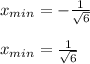
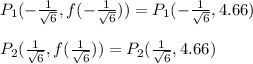
(these are the point in which the function change of a decrease to an increase)

The same reason as before. There in one local maximum:

`x_(max)=0`

`P(0,f(0))=P(0,5)`

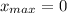
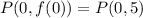
(c) The inflection points are calculated by using the second derivative:

`(d^2f)/(dx^2)=144x^2-8=0\\\\x^2=(8)/(144)=(1)/(18)\\\\x=\pm (1)/(√(18))`

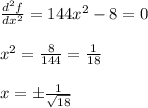
Then , there are two inflection points , given by:

`P_1(-(1)/(√(18)),f(-(1)/(√(18))))=P_1(-(1)/(√(18)),4.82)\\\\P_2((1)/(√(18)),f((1)/(√(18))))=P_2((1)/(√(18)),4.82)`