Answer:
For this case we know that the total distance to travel is 325 mi. And we know that Aretha travels at the following velocity:
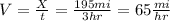
Then we can use the following definition:
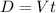
Where D is the distance and V the velocity. And solving for t we got:
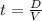
And replacing we got:
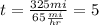
So then we can conclude that after 5 hours at this rate Aretha will arrive to home
Explanation:
For this case we know that the total distance to travel is 325 mi. And we know that Aretha travels at the following velocity:
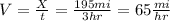
Then we can use the following definition:
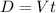
Where D is the distance and V the velocity. And solving for t we got:
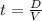
And replacing we got:
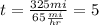
So then we can conclude that after 5 hours at this rate Aretha will arrive to home