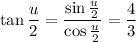Question

Find the exact values of sin(u/2) cos(u/2) and tan(u/2) given sin u=24/25 and pie/2 < u < pie

Answer 1

Recall the double/half angle formulas:

`\cos^2\frac x2=\frac{1+\cos x}2`

`\sin^2\frac x2=\frac{1-\cos x}2`

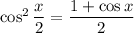
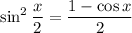
We're given
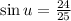`\sin u=(24)/(25)`, and since
`u` is between π/2 and π, we expect
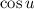`\cos u` to be negative. So from the Pythagorean identity, we find

`\sin^2u+\cos^2u=1\implies\cos u=-√(1-\sin^2u)=-\frac7{25}`

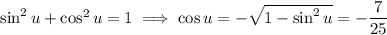
Also, we know
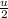`\frac u2` will fall between π/4 and π/2, so both
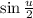`\sin\frac u2` and
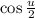`\cos\frac u2` will be positive. Then we find

`\cos\frac u2=\sqrt{\frac{1+\cos u}2}=\frac35`

`\sin\frac u2=\sqrt{\frac{1-\cos u}2}=\frac45`

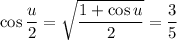
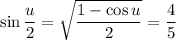
and it follows that

`\tan\frac u2=(\sin\frac u2)/(\cos\frac u2)=\frac43`