Answer:
1.9
Explanation:
Given the cups of coffee drunk every day over a r]year represented by the probability distribution.
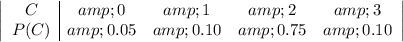
The mean number of coffee is the expected value of the probability distribution table above.
Expected Value,
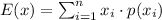
Therefore:
E(C)=(0X0.05)+(1X0.10)+(2X0.75)+(3X0.10)
=0+0.10+1.5+0.3
Expected Value=1.9
Therefore, the mean number of coffee sold =1.9