Answer:
The correct answer is option 1.
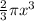 cubic units.
cubic units.
Explanation:
The formula for the volume, V of a right angled cone is given as:
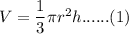
Where,
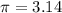
 is the radius of the circular base of the cone.
is the radius of the circular base of the cone.
 is the height of the cone.
is the height of the cone.
We are given that height is twice of the radius of its base.
Let the radius of base =
 units
units
Now, As per the question statement,
The height of cone=
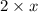 units
units
Putting the values of
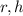 in equation (1) to find the volume:
in equation (1) to find the volume:
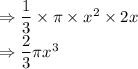
Hence, the correct answer is option 1.
 cubic units.
cubic units.