Explanation:
Take the natural log of both sides:
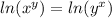
Logarithm rules allow you to bring down the exponents:
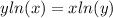
Now differentiate. We will have to implicitly differentiate 'y' since it is a function of 'x'. Both sides require the product rule:
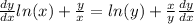
Isolate the terms that have y' since that is what we want:
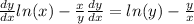
Factor out y' to get:
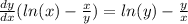
Therefore:
