Question

What is the surface area of the solid that this net can form?

106 square centimeters
150 square centimeters
210 square centimeters
270 square centimeters
((PLEASE HELP 36 MINUTES LEFT))​

Answer 1

C. 210 square centimeters.

Explanation:

The figure is attached.

Notice that the whole surface area is formed by 1 square, 2 different rectangles and 2 conguent triangles. Let's find the area of each figure.

Square area:

`A_(square) =(5cm)^(2) =25 \ cm^(2)`

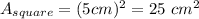
Rectangles:

`A_(r1)=13cm * 5 cm=65 \ cm^(2)`

`A_(r2)= 12cm * 5cm= 60 \ cm^(2)`

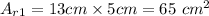
So, all rectangles have a total surface area of
`A_(rectangles)=65+60=125 \ cm^(2)`

Triangles:

`A_(triangles)=2((1)/(2) * 12cm * 5cm ) =60 \cm^(2)`

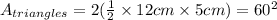
Because, there are two congruent triangle.

Now, we need to sum all areas:

`S=25+125+60=210 \ cm^(2)`

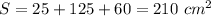
Therefore, the total surface area is 210 square centimeters. So, the right answer is C.