Question

Which expression is equivalent to log Subscript 12 Baseline StartFraction x Superscript 4 Baseline StartRoot x cubed minus 2 EndRoot Over (x + 1) Superscript 5 Baseline EndFraction?

4 log Subscript 12 Baseline x + one-half log Subscript 12 Baseline (x cubed minus 2) minus 5 log Subscript 12 Baseline (x times 1)


4 log Subscript 12 Baseline x + one-half log Subscript 12 Baseline StartFraction x cubed Over 2 EndFraction minus 5 log Subscript 12 Baseline 1


log Subscript 12 Baseline 4 x + one-half log Subscript 12 Baseline (x cubed minus 2) minus 5 log Subscript 12 Baseline (x) + 1


4 log Subscript 12 Baseline x + one-half log Subscript 12 Baseline (x cubed minus 2) minus 5 log Subscript 12 Baseline (x + 1)

Answer 1

D

4logw (x^{2} - 6) - 1/3logw (x^{2} + 8)

Explanation:

Answer 2

(D)
`4log_(12)x+(1)/(2) log_(12)(x^3-2)-5log_(12)(x+1)`

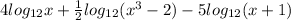
4 log Subscript 12 Baseline x + one-half log Subscript 12 Baseline (x cubed minus 2) minus 5 log Subscript 12 Baseline (x + 1)

Explanation:

Given the expression:

`log_(12)(x^4√(x^3-2) )/((x+1)^5)`

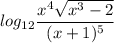
We first apply the division law of logarithm:
`log_(a)x/y=log_(a)x-log_(a)y`

`log_(12)(x^4√(x^3-2) )/((x+1)^5)=log_(12)x^4√(x^3-2)-log_(12)(x+1)^5`

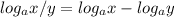
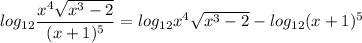
Next, by addition law:
`log_(a)xy=log_(a)x+log_(a)y`

`=log_(12)x^4+log_(12)√(x^3-2)-log_(12)(x+1)^5\\\\Log a^m=mLog a, Log √(x)=log x^(1/2)\\\\ =4log_(12)x+log_(12)(x^3-2)^(1/2)-5log_(12)(x+1)\\\\=4log_(12)x+(1)/(2) log_(12)(x^3-2)-5log_(12)(x+1)`

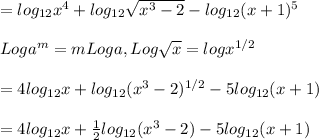
The correct option is D.