Question

A large tank of height h= 1 m and diameter D = 0.75 m is fixed on a cart, as shown in the sketch below. Water exits from the tank through a nozzle of diameter d = 15 mm. The speed of the liquid leaving the tank is approximately: V = V2gy, where y is the height from the nozzle to the free surface. Determine the tension in the wire when y = 0.9 m. A) Plot the tension in the wire as a function of water depth for: 0 < y < 0.9

Answer 1

the tension in the wire =
`3.12 \ N |_{y= 0.9 \ m`

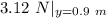
Step-by-step explanation:

Parameters given include:

The height of the large tank = 1 m

Diameter D = 0.75 m

diameter of the nozzle where the water exit d = 15 mm = 0.015 m

The flow speed at the exit of the tank
`\mathbf{V = √(2gy) }`

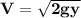
The first diagram shown in the attached file depicts and illustrate the sketch of the large tank that is fixed on the cart.

Now; using the x component of the momentum equation from the diagram; we have;

`{ \bar F_(xx) } + { \bar F_(bx) } = (\delta )/(\delta t)\int\limits_(cv) \bar u pd. V + \int\limits_(cs) \bar u pV. A`

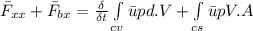
For steady flow:

`(\delta )/(\delta t)= 0`

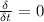
So:

T = u{║ρV₁A₁║}

T = ρV₁²A₁

where:

`\mathbf{V = √(2gy) }`

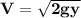
T =ρgy
`(\pi d^2)/(2)`

replacing y= 0.9 m

The tension of the wire is:

T = 999 × 9.81 × 0.9 ×
`(\pi *0.015^2)/(2)`

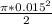
T =
`3.12 \ N |_{y= 0.9 \ m`

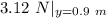
Hence, the tension in the wire =
`3.12 \ N |_{y= 0.9 \ m`

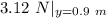
The schematic graphical representation showing the plot of the tension in the wire as a function of water depth for: 0 < y < 0.9 can be found in the document file attached below.